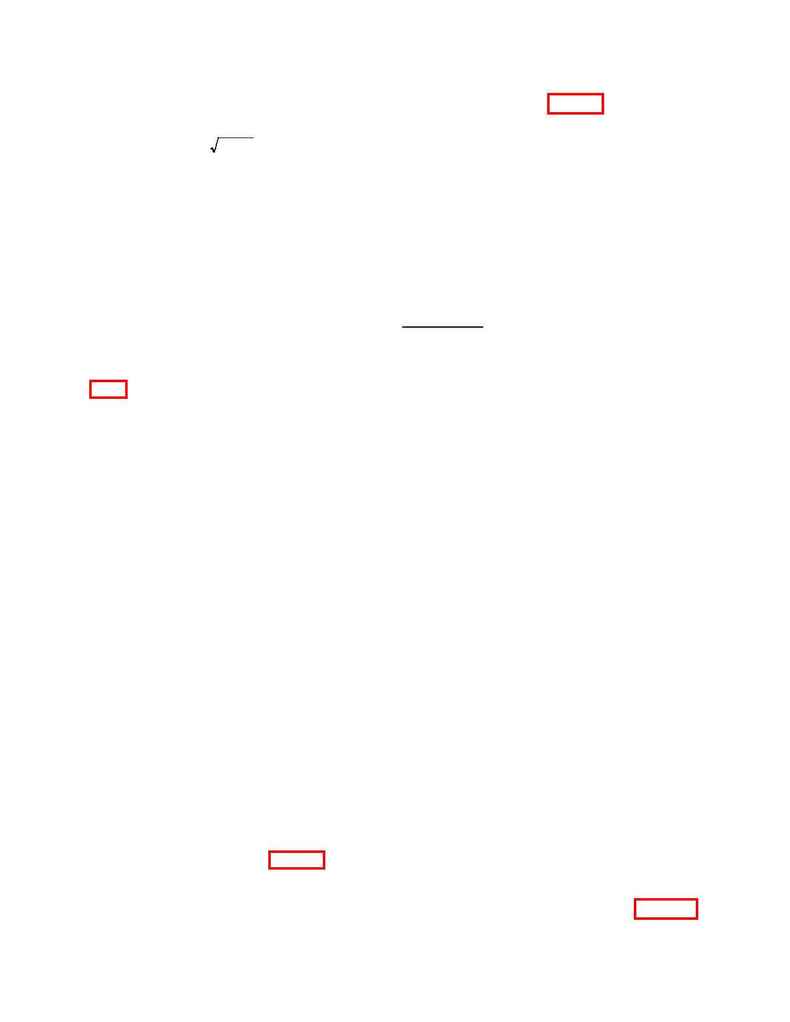
fit. We also calculated the skewness S of the
distribution (all data included), finding S/
S
=
2.6, where
is the theoretical
r
S
=
15/N
standard deviation for the skewness of an ideal
Gaussian distribution. It is difficult to interpret
the significance of a nonzero skewness, since it
is highly sensitive to the tail, which in turn suf-
fers from small number statistics. A value 2.6
standard deviations from the expected is possi-
bly significant.
The nonzero skewness of our distribution
arises almost entirely from the presence of the
four orbits making up the bump near 1.4 in Fig-
ure 4. Removing these orbits drops the skew-
ness to approximately one standard deviation
from a perfect Gaussian. Are these four orbits
anomalous, or do they just represent statistical
fluctuations? One can ask this question: given
the expected number of orbits in a particular
bin, what is the probability of finding the
observed number? One may also adjust bin
width to gauge sensitivity to bin boundary
placement. For the bump orbits, that probabil-
ity ranges, depending on bin boundaries, from
six to eighteen percent not unreasonably
small, and relatively insensitive to bin width.
Nevertheless, we reintegrated these orbits with
microscopically different initial conditions and
found that they shifted out of the tail, removing
the bump. As a further check, we then reinte-
grated seven other orbits chosen at random
from the distribution and observed the same
kind of movement within the distribution.
Thus, we conclude that the noise in T
L
is such
G
boundaries of the Gaussian fit. The standard
deviation of this distribution is almost a factor
of three smaller than the
calculated from the
LFM data involving Jupiter at its actual mass.
Thus, the distribution width is a function of
mass ratio. It is also apparent from the figures
in LFM that
is an increasing function of
orbital inclination of the test particle.
Summary
We have examined the 25 "non-resonant"
outer-belt asteroids in light of the T
L
-T
e
relation
and found that their predicted event times,
though significantly less than the age of the
solar system T
SS
, are statistically consistent
with their being present today. The key to this
conclusion is that chaotic orbits are, to a close
approximation, normally distributed about the
mean T
L
-T
e
relation for the particular mass
ratio and dynamical configuration in question.
We think that the 25 objects are the expected
distribution tail of an originally much larger
population, which has been thinned out by Jupi-
ter. The adjustable parameters of the T
L
-T
e
relation are relatively insensitive to mass ratio
and dynamical configuration. However, the
distribution width crucial for interpreting the
significance of predicted event times is a
function of the mass ratio. We have noted the
existence of one such misinterpretation in the
recent literature, and we urge caution to the
dynamical community to prevent such mistakes
in the future.
The two facets of the problem discussed in
Chaotic Motion in the Outer Asteroid Belt
page 12
