Astronomical Applications Department, U.S. Naval Observatory Precession Maple Page 25
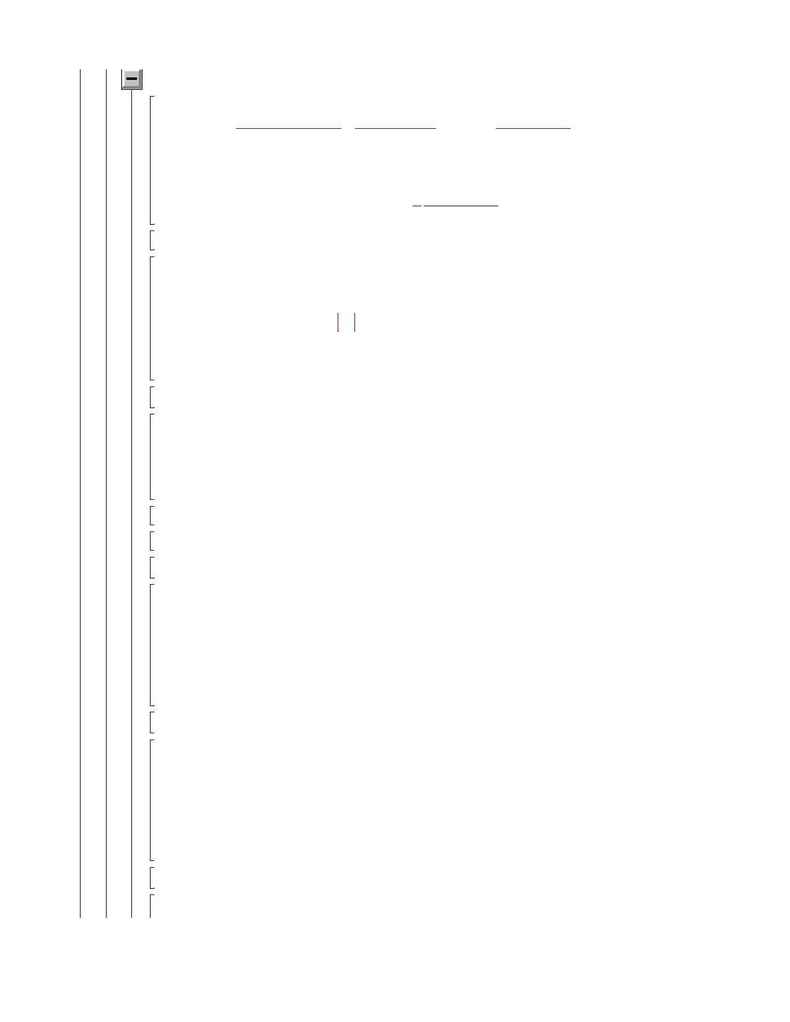
Conversion of the Torque Integral to the Body Frame
Define
=
B
1
-
(
)
+
b
a
( )
cos
U
2
( )
sin
2
+
+
b
2
b a
a
2
3
( )
sin
2
and
=
B
2
(
)
+
b
a U Q
2
( )
sin
. Then the torque
integral becomes
=
B
2
1
2
(
)
+
a
b U Q
( )
sin
:=
Bsubs
[
]
,
%% %
=
K
K
K
d
0
2
(
)
- +
a
b
( )
cos
P
-
B
2
-
+
+
2 B
1
A
C
( )
cos
B
2
B
1
Q
-
B
1
Q
Check:
(
)
map
,
simplify
(
)
evalm
-
(
)
subs
,
Bsubs
(
)
op
,
[
]
,
2 1
%
(
)
op
,
[
]
,
2 1
torque_cone
0
0
0
:=
torque_cone
%%
(
)
latex
,
torque_cone "d:/dynamics/precession/ConeTorqueIntegralCone.tex"
The pressure components in the cartesian body frame are
=
(
)
mat
,
,
x
y
z
(
)
' '
R
,
,
&*
(
)
mat
,
,
X
Y
Z
=
x
y
z
(
)
R
,
,
&*
X
Y
Z
Similarly, the conversion from the cartesian body frame to the conical body frame is
=
(
)
mat
,
,
(
)
CartToCon
,
&*
(
)
mat
,
,
x
y
z
=
( )
sin
( )
cos
( )
sin
( )
sin
-
( )
cos
-
( )
sin
( )
cos
0
( )
cos
( )
cos
( )
cos
( )
sin
( )
sin
&*
x
y
z
Hence, we have
(
)
subsop
,
=
[
]
,
2 2
(
)
rhs '
'
%%
%
Page 25
