"longitudinal" gradients, where the temperature gradient is in the direction from the CMA to the
primary (parallel to the primary mirror axis of symmetry), and "transverse" gradients, which are
perpendicular to the longitudinal gradients. Both cases assume, as a worst-case scenario, that the
gradient direction lies in the plane of the optical bench.
For convenience, I summarize here the calculations that follow in Sections 2 and 3. I find that
the change in basic angle
due to the imposition of a gradient
is
dy
= -
2a
cos
2
x c
È
+
O
(
c
È
2
)
for the longitudinal case and
dy
=
2
h
+
a tan x
cos
2
x c
Ç
+
O
(
c
Ç
2
)
for the transverse case. Here,
, where
is the CTE of the material,
is the angle of the
c h a $ b
CMA mirrors with respect to the primary mirror symmetry axis,
h is the distance along the sym-
metry axis from the CMA support(s) to the CMA mirrors, and 2a is the width of the CMA block
(same as the primary mirror width, currently 60 cm). (cf. Figures 1, 3, and 6.) These equations
impose the temperature gradient constraints
b
È
[
cos
2
x
2a a t
and
Basic Angle Temperature Gradient Sensitivity
FTM-USNO-95-01
2
5
10
15
20
25
30
35
40
45
50
1
1.5
2
2.5
3
3.5
4
4.5
5
14
13
12
11
10
10
9
9
8
8
7
7
6
6
5
5
5
4
4
4
3
3
3
2
2
1
1
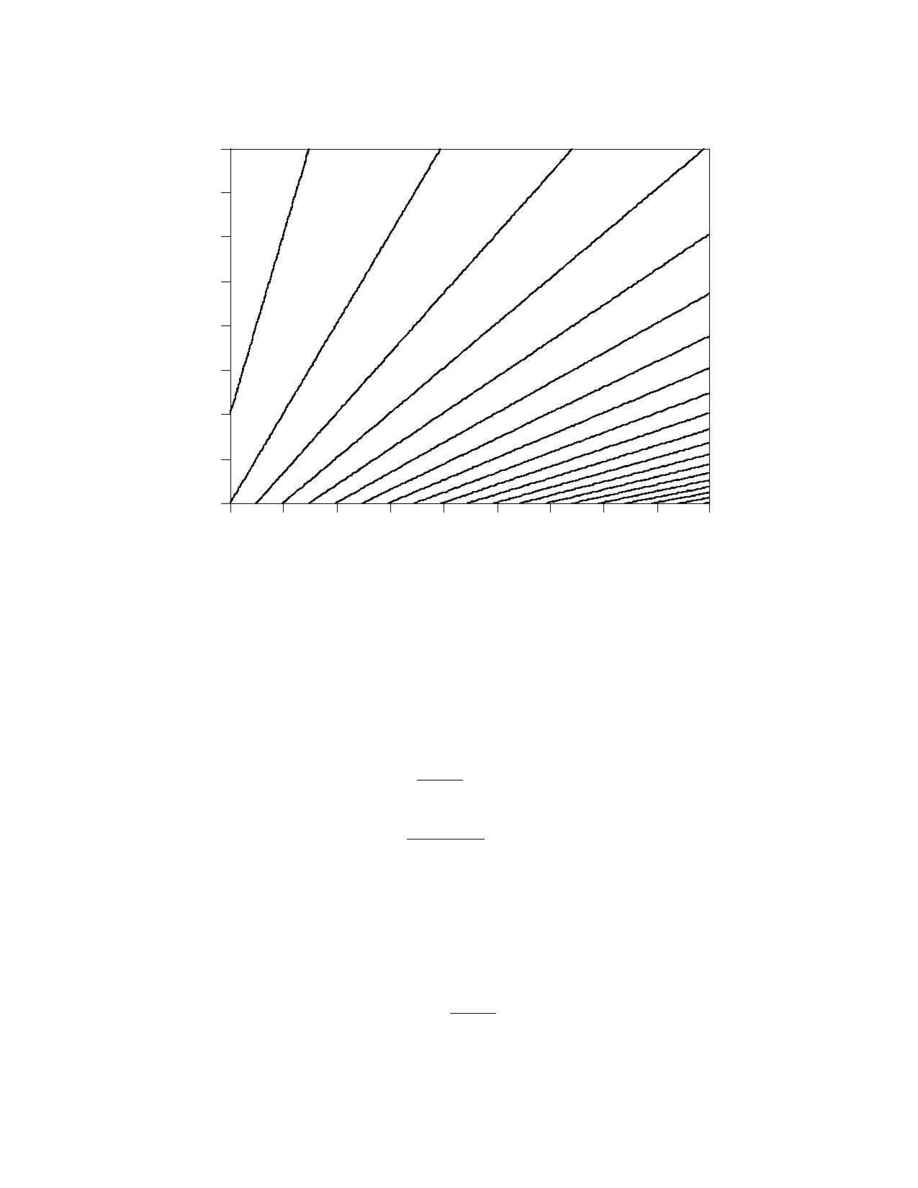
Figure 2
(10
-8
K
-1
)
(mK/m)
(
µ
as)
