(31)
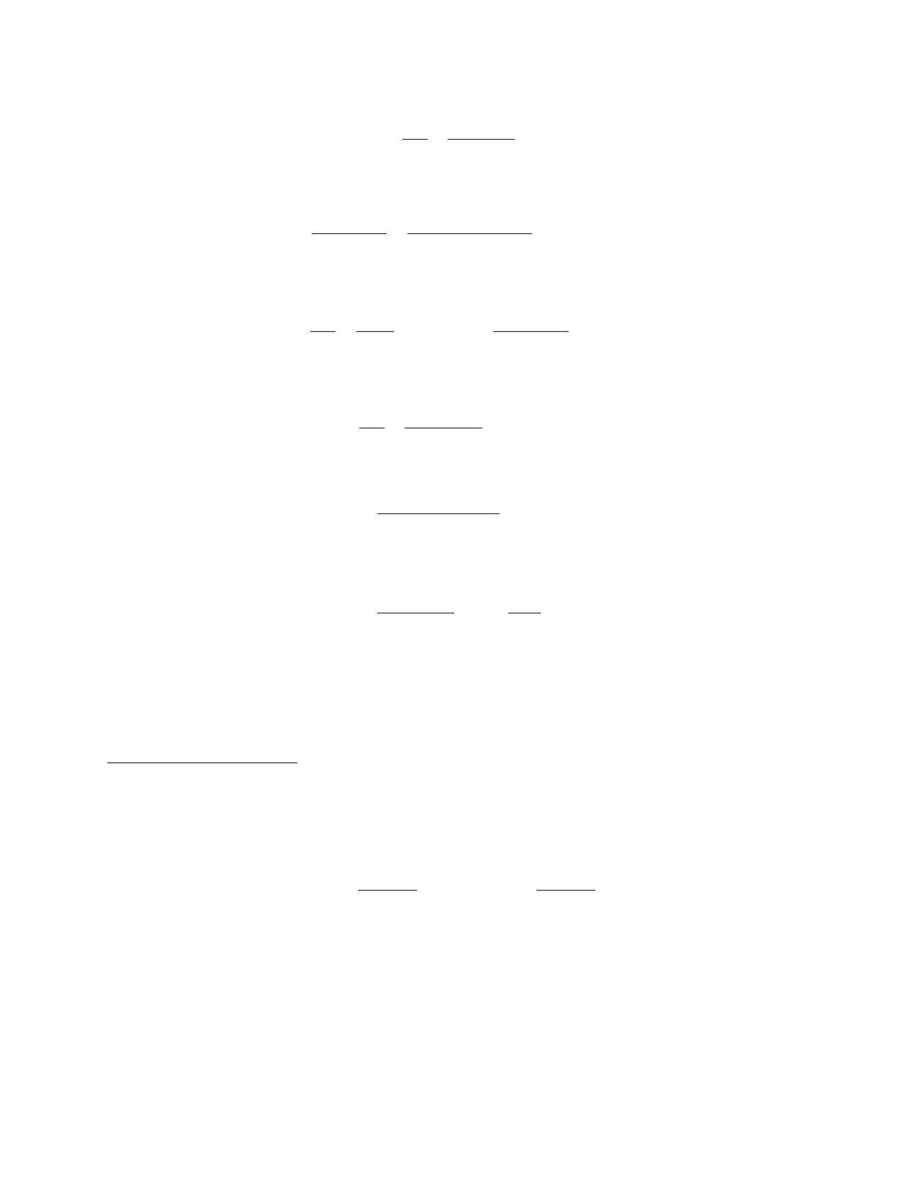
dy
2
=
Ds
z, y
2a
Now integrate
s across the mirror. The slope of the tangent along the z direction is
(32)
╣Ds
z, y
╣z
=
h
+
(2a
-
z) tan x
cos
2
x
c
+
O
c
2
The change in angle, averaged over the 2a by 2b plane, is then
(33)
dy
2
=
1
4 a b
░
0
2a
░
-
b
b
w
z, y
╣Ds
z, y
╣z
dy dz
Evaluating (33) with w(z,y) = 1, we have simply
(34)
dy
2
=
h
+
a tan x
cos
2
x c
+
O
c
2
Solving for
, we find that the temperature gradient constraint is
(35)
b [
cos
2
x
2 a
h
+
a tan x
t
+
O(t
2
)
For the case h = 0,
,
,
, and
, we have
x
=
45 deg t
=
25 lrad a
=
30 cm
a
=
2 $ 10
-
8
K
-
1
cos
2
x
2 a a tan x t
=
5.1 mK
m
The tolerance on the transverse temperature gradient due to a constraint on the basic angle devia
-
tion is similar to that of the longitudinal gradient, differing by a factor and, more impor-
tan x
tantly, by a dependence on the distance
h.
3.3. Beam Divergence.
The curvature of the mirrors introduced by a transverse temperature gradient will cause the colli
-
mated input beam to diverge. Using eq. (16) to calculate the radius of curvature for the surface
function given by eq. (30), we find
(36)
f
a
=
-
cos
2
x
2c tan x
and
f
b
= -
1
2c tan x
where f
a
and f
b
are again the focal lengths along the long and short dimensions. Note the similari
-
ties to eqs. (17). A CTE of
and a temperature gradient
= 5 mK/m again pro-
a
=
2 $ 10
-
8
K
-
1
duce focal lengths of 2.5 and 5.0 gigameters, since I'm using
= 45 deg. The conclusions of
section 2.4 also hold for the transverse case.
Basic Angle Temperature Gradient Sensitivity
FTM-USNO-95-01
10
