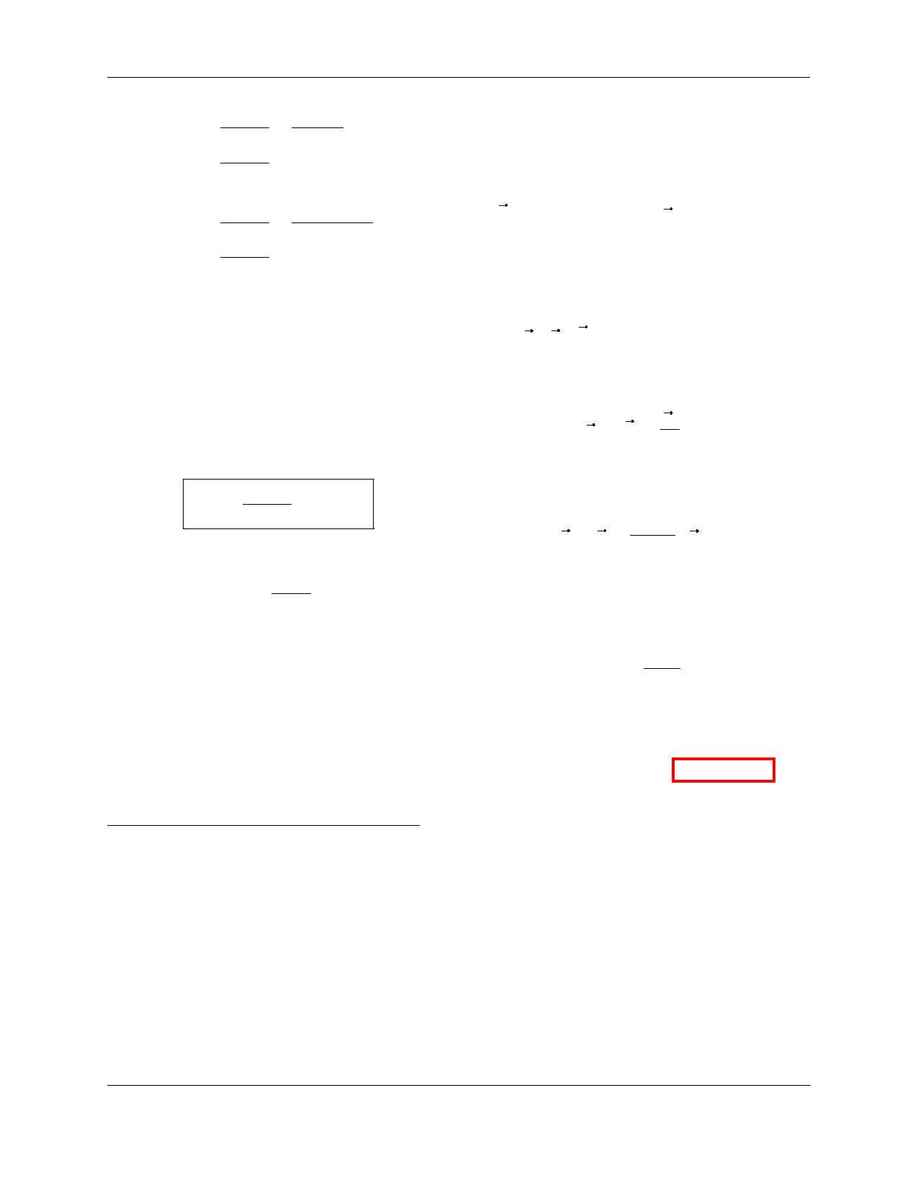
(6)
k
2
=
1
t
2
-
t
0
ln
d
2
$ a
1
d
0
=
1
t
2
-
t
0
ln(a
1
$ a
2
)
k
3
=
1
t
3
-
t
0
ln
d
3
$ a
2
$ a
1
d
0
=
1
t
3
-
t
0
ln(a
1
$ a
2
$ a
3
)
®
and so on. The multiplicative factors
are derived in section 3, in
a
1
, a
1
$ a
2
,
¬
case it is not intuitively obvious. We there-
fore conclude that the instantaneous Lyapu-
nov exponent is
(7)
k
n
=
1
t
n
-
t
0
S
i
=
1
n
ln a
i
where we have defined
(8)
a
i
h
d
(
t
i
)
d
(
t
0
)
As long as the rescalings take place in the
linear regime, this construction is valid.
Notice that, in a computer, only the accumu-
lating sum of the natural log of the
i
need
be stored. In addition, the time intervals
need not be evenly spaced.
2. Renormalization of the Test Orbit.
The rescaling of the test particle orbit is per-
formed on the test - reference phase space
distance vector. Whenever the distance d(t)
becomes greater than or equal to the thresh-
old D, we scale the test particle distance
from the reference particle by the factor 1/
i
,
maintaining the current relative orientation
between the two particles in phase space.
Write the reference and test particle phase
space vectors as
(9)
R h
x
y
z
v
x
v
y
v
z
ref
and r h
x
y
z
v
x
v
y
v
z
test
Define
. Then the adjustment to
q
q h r
-
R
the test particle phase space coordinates at
time t
i
is
(10)
r
i
b R
i
+
q
q
i
a
i
Alternatively, one could write the equivalent
expression
(11)
r
i
b r
i
-
a
i
-
1
a
i
$ qq
i
Eq. (10) is slightly less expensive to calcu-
late than eq. (11). All we are doing is res-
caling the distance d(t),
(12)
d
(
t
i
)
b
d
(
t
i
)
a
i
in the appropriate direction in phase space.
I have found that
and
d
0
=
10
-
6
D
=
10
-
4
0
ranging from 10
-5
to
10
-15
, with a rescaling threshold of 10
-4
. One
can see that values of d
0
in the range 10
-5
to
10
-8
are adequate. Smaller than this invites
numerical trouble due to the finite word size
of the machine.
I have also found that any difference
between using the full phase space distance
f:\dynamics\text\lyapcalc.lwp
Maximum Lyapunov Exponent Calculation
7:07pm September 30, 1995
2
